The initial speed of an arrow is 15m/s at an elevation of 30°. What is its speed at the highest point of its trajectory?
Answers 2
Answer:
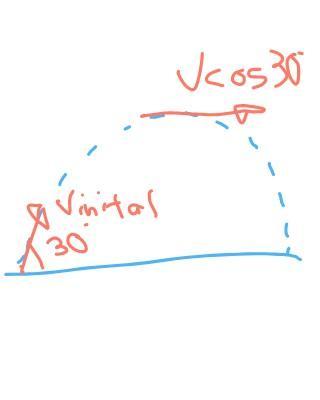
At highest point vertical component of initial velocity becomes zero so at highest point the velocity of arrow will be
Vᵃᵗ ʰⁱᵍʰᵉˢᵗ = Vⁱⁿⁱᵗⁱᵃˡcosθ
= 15× cos30
= 15 × √3/2
= 6.99 ≈ 7 m/s
-
Author:
cassiemduz
-
Rate an answer:
2
- Initial speed of arrow is 15 m/s.
- Angle of projection = 30°.
- Velocity at highest point?
- This is an example of GROUND-GROUND PROJECTILE.
We know that , acceleration due to gravity is applicable only along Y axis , hence the velocity component along X axis will always remain constant.
At highest point, the projectile only has the X component velocity (because Y component becomes zero).
[tex]v_{highest} = v \cos( \theta) [/tex]
[tex] \implies v_{highest} = 15 \times \cos( {30}^{ \circ} ) [/tex]
[tex] \implies v_{highest} = 15 \times \dfrac{ \sqrt{3} }{2} [/tex]
[tex] \implies v_{highest} = 12.99 \: m {s}^{ - 1} [/tex]
So, velocity at max height is 12.99 m/s
-
Author:
paitynhendrix
-
Rate an answer:
3
