a swimmer can swim at a speed of 0.6m/s with respect to water . she wants to cross a river which is 50 m wide and has a water current of 0.36 m/s . if she wants to reach on the other bank of the point directly opposite to her starting point in which direction she must swim
-
Subject:
Physics -
Author:
adalynmontoya -
Created:
1 year ago
Answers 2
Answer:
here is your answer hope it helps
-
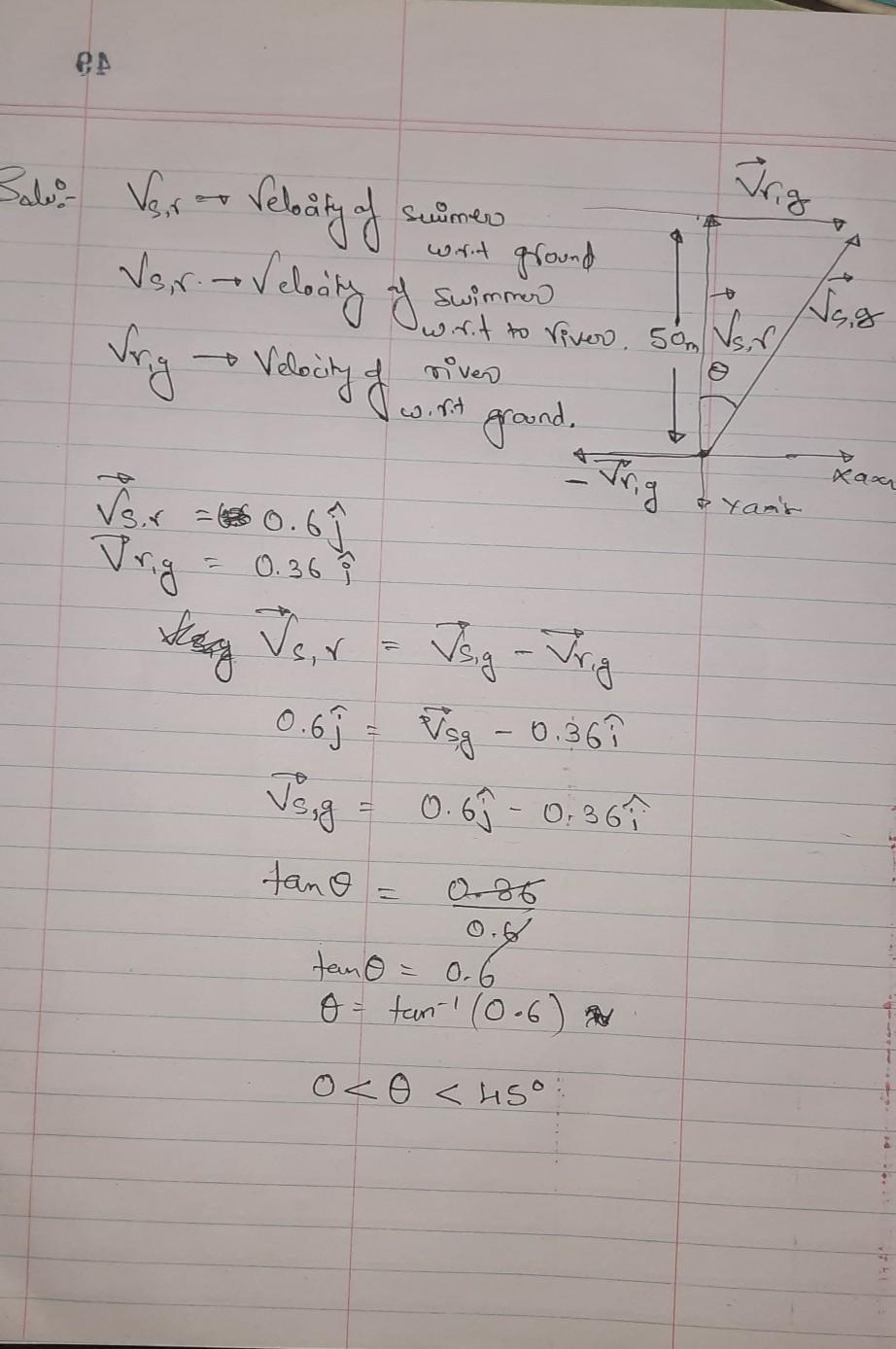
Author:
winniehv4w
-
Rate an answer:
7
Given:
Speed of swimmer [tex]V_{s}=0.6 m/s[/tex]
Width of the river [tex]=50m[/tex]
Speed of the water in river [tex]V_{w} =0.36m/s[/tex]
To find:
The direction in which he swimmer should swim in order to reach the exact opposite point.
Solution:
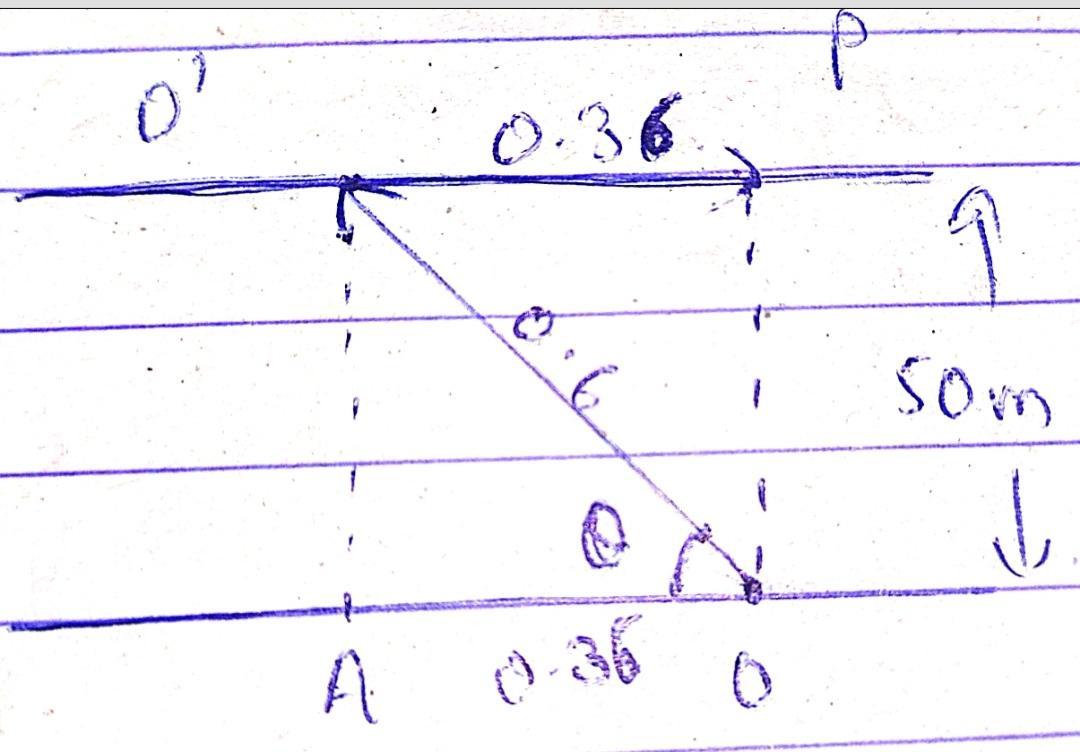
From the figure, lets assume that the swimmer starts swimming from [tex]O[/tex] with a speed [tex]V_{s}=0.6m/s[/tex]. According to the question, the swimmer wants to reach the point [tex]P[/tex] but, as we know, the river is flowing with a velocity [tex]V_{w}=0.36m/s[/tex].
If the swimmer swims in the direction [tex]OP[/tex], he will lose the trajectory of the path and miss the point [tex]P[/tex] by flowing in the direction of the river.
To cover this gap, the swimmer needs to swim in the direction opposite to the flow of the river at an angle θ with the horizontal.
Lets assume, the target point of the swimmer be the point [tex]O'[/tex] which makes an angle θ with the river. Hence, with the presence of the flow of river, swimmer reaches the required point [tex]P[/tex].
Now,
In Δ[tex]OAO'[/tex], we have, ∠[tex]AOO'=[/tex] θ, Hence,
[tex]cos[/tex]θ [tex]=\frac{OA}{O'O}[/tex]
[tex]cos[/tex]θ [tex]=\frac{0.36}{0.6}[/tex]
[tex]cos[/tex]θ [tex]=0.6[/tex]
Hence,
θ [tex]=cos^{-1}(0.6)[/tex]
θ [tex]=53^{o}[/tex]
The swimmer needs to travel with an angle of [tex]53^{o}[/tex] with respect to the origin with the horizontal.
Final answer:
Hence, the swimmer should travel in the direction opposite to the flow of river at an angle of 53° with respect to the horizontal.
-
Author:
midnightkeith
-
Rate an answer:
12
If you know the answer add it here!
Other related questions that may help you
-
Author:
zion2
-
Author:
bebejames
-
Rate an answer:
3
-
Author:
carlosgutierrez
-
Rate an answer:
19
-
Author:
mr kitty
-
Author:
honey piea6nf
-
Rate an answer:
18
-
Author:
flyby
-
Author:
little bear5biv
-
Rate an answer:
5
-
Author:
lauran3oq
-
Rate an answer:
4