Solution set of x^2-9x+18>0 is

Answers 1

EXPLANATION.

Solution set : x² - 9x + 18 > 0.

As we know that,

Factorizes the equation into middle term splits, we get.

⇒ x² - 6x - 3x + 18 > 0.

⇒ x(x - 6) - 3(x - 6) > 0.

⇒ (x - 3)(x - 6) > 0.

Now, we find zeroes of the expression, we get.

⇒ (x - 3) = 0.

⇒ x = 3.

⇒ (x - 6) = 0.

⇒ x = 6.

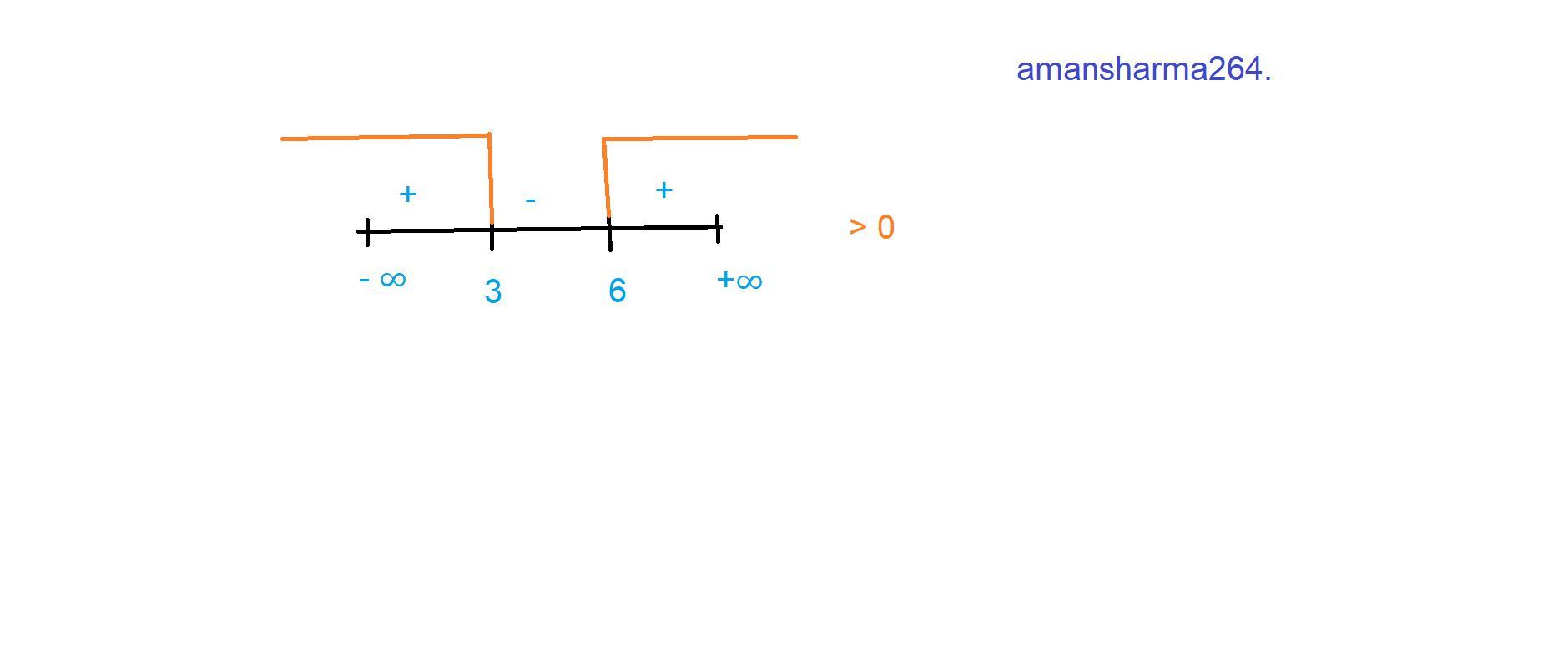
Now, we put this point on wavy curve method, we get.

⇒ x ∈ (- ∞, 3) ∪ (6, ∞).

MORE INFORMATION.

Rules of inequality.

We can multiply Or divide any non - zero number "k" on both sides of inequality sign of inequality will change according to sign of k that is,

If k > 0 then sign of inequality will remain same.

If k < 0 then sign of inequality will get reversed.

Wavy curve method.

Step - 1 : Check structure.

Step - 2 : Find critical points.

Step - 3 : Plot all critical points on number lines.

Step - 4 : Check sign of given example by putting value of x from different parts of number line.