Answers 2
[tex]\huge\underline\mathcal{\red{A}\blue{n}\pink{s}\purple{w}\orange{e}\green{r} -}[/tex]
- To do - verify the identity a³ + b³ = ( a + b )( a² - ab + b² )
The stated can be verified as follows ~
[tex]a {}^{3} + b {}^{3} = (a + b)(a {}^{2} - ab + b {}^{2} )[/tex]
LHS = a³ + b³
RHS = ( a + b )( a² - ab + b² )
Let's solve the RHS part in order to verify this !
[tex](a + b)(a {}^{2} - ab + b {}^{2} ) \\ \\ \longrightarrow \: a {}^{3} - \cancel{a {}^{2} b }+ \cancel{ab {}^{2}} +\cancel{ a {}^{2} b }- \cancel{ab {}^{2} } + b {}^{3} \\ \\ \longrightarrow a {}^{3} + b {}^{3} = LHS[/tex]
Hence , verified !
hope helpful ~
-
Author:
eaglegk9n
-
Rate an answer:
10
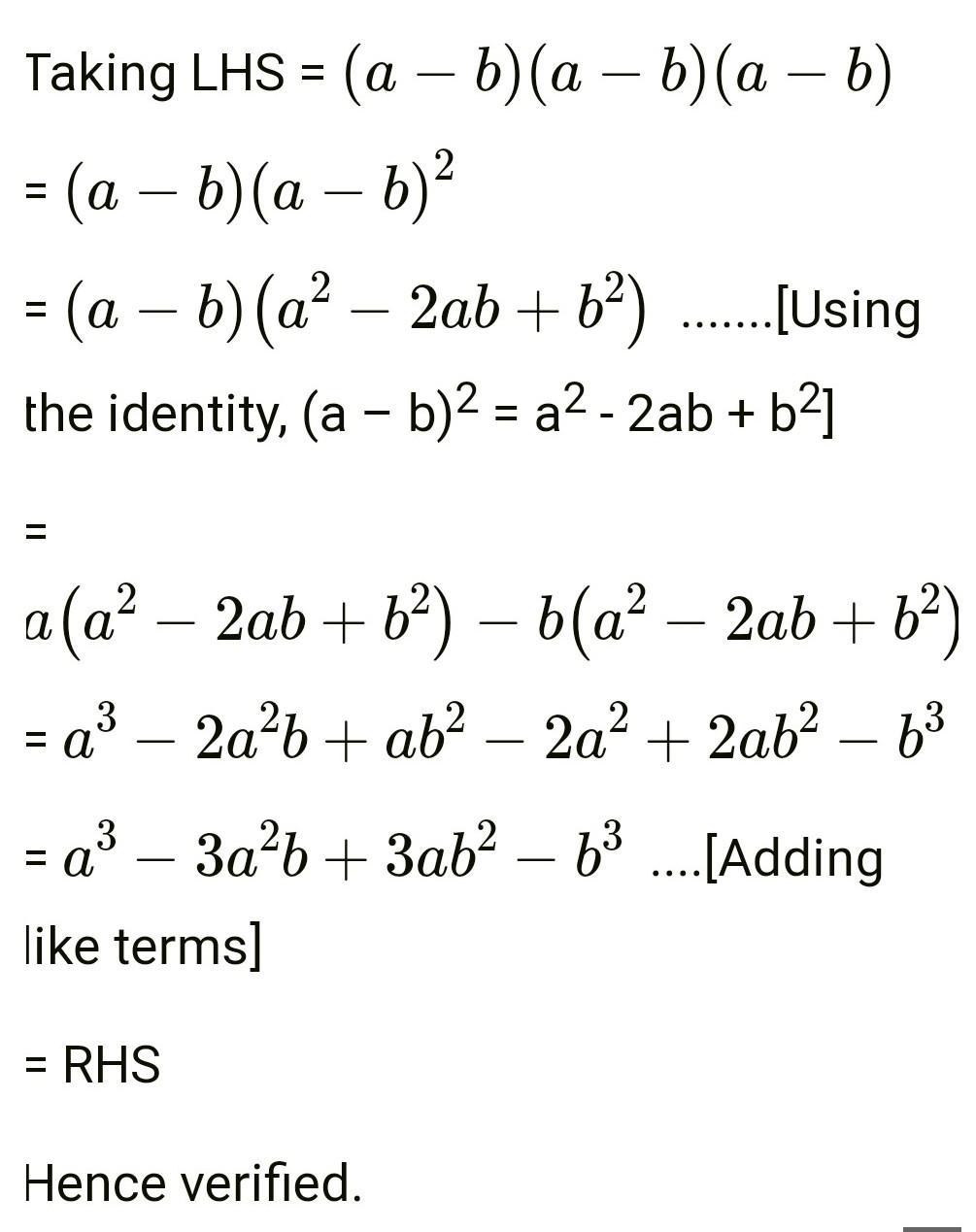
Step-by-step explanation:
Hope you're satisfied with my answer.....
-
Author:
franciscahoffman
-
Rate an answer:
2
If you know the answer add it here!
Choose a language and a region
How much to ban the user?
1 hour
1 day
100 years
