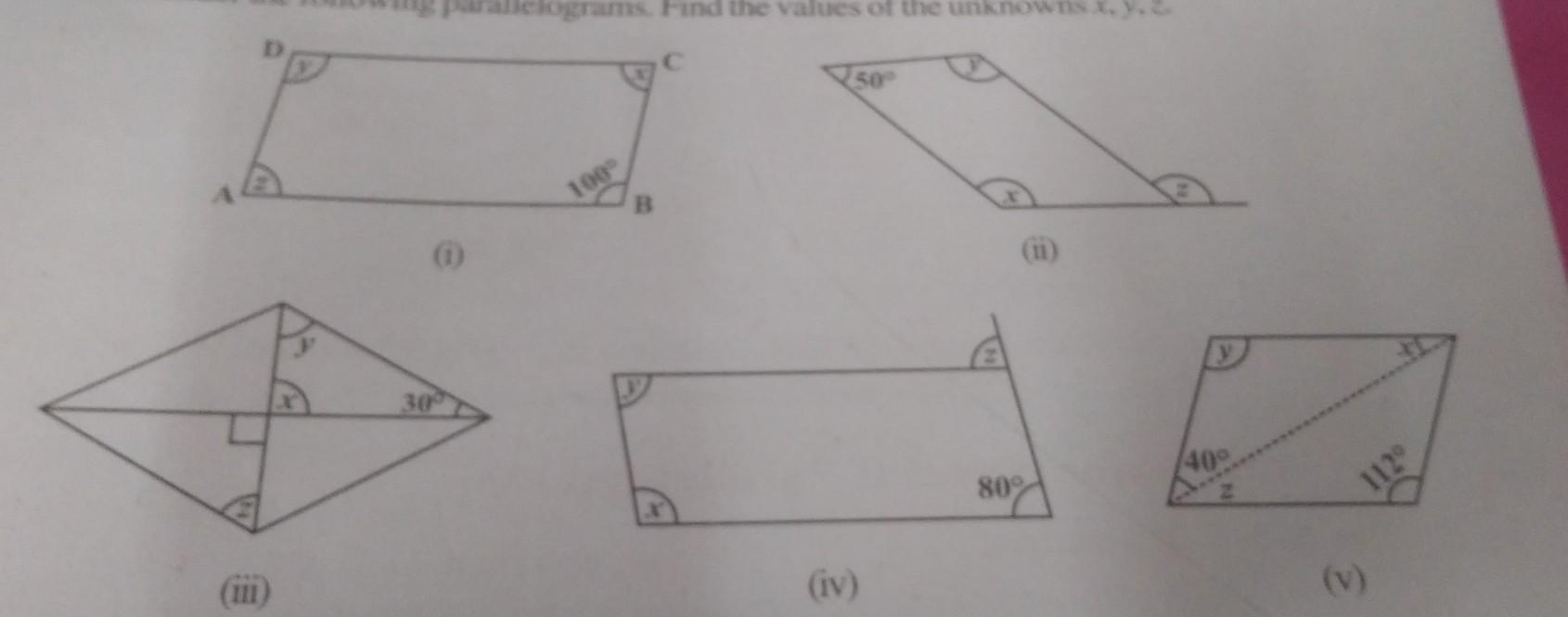
Consider the following parallelograms. Find the values of the unknowns x. y. z.
-
Subject:
Math -
Author:
martínmgvu -
Created:
1 year ago
Answers 2
-
Author:
coffey
-
Rate an answer:
1
Before moving forward, I'll take parallelogram as pg (to make it shorter) and here are some important points:
- Opposite angles of a pg are equal
- Adjacent angles (angles lying on the same line) are supplementary
- Linear pairs are equal
- Sum of all angles of a triangle will be always 180°
in parallelogram i:
We know that opp. angles of a parallelogram are equal.
So, y = 100
= 100°
Also, adjacent angles of a pg are supplementary (one angle will be 180 - other angle)
So, z = 180 - 100
= 180-100
= 80°
Since opposite angles are equal, x = z = 80°
In parallelogram ii)
z = 50° (corresponding angles equal)
y = 180 - 50 = 130°
x = y = 130°
In parallelogram iii)
Here in the case of z, there's a slight trick. as we learned earlier, adjacent angles are supplementary. The same applies here but make sure to multiply 30° by 2 and divide z by 2 since the angle is divided into 2 equal parts by the diagonal of the pg.
= 30 x 2 = 60°
z = 180-60/2
= 120 / 2
= 60°
Since opp. angles are equal, z = y
y = z = 60°
To find x, just apply the triangle angle rule. As we know, the sum of all angles will be 180°. So,
y + 30 + x = 180
60 + 30 + x = 180
90 + x = 180
∴ x = 180 - 90 = 90°
In parallelogram iv)
x = 180 - 80
= 100°
y = 80°
z = 80° (linear pair)
In parallelogram v)
x = 40°
z = 40° (2 × x)
y = 112°
This answer ends here. Hope it helped! If it helped, then pls mark it as brainliest! Thanks and have a nice day.
-
Author:
lillianti9b
-
Rate an answer:
10
