The product of two 2-digit numbers is 535 and their GCD is 7. Find their LCM.
Answers 2
Answer:
57
Step-by-step explanation:
search-icon-header
Search for questions & chapters
search-icon-image
Class 10
>>Maths
>>Quadratic Equations
>>Solutions of Quadratic Equations
>>A two digit number is such that the prod
Question
Bookmark
A two digit number is such that the product of the digits is 35, when 18 is added to the number, the digits interchange their places. Find the number.
Medium
Solution
verified
Verified by Toppr
Correct option is A)
Solution:-
Let the two digit no. be xy.
Then its decimal expansion becomes 10×x+y
When digits are interchanged, the number is yx.
Then its decimal expansion becomes 10×y+x
x×y=35{Given}
⇒x=
y
35
⟶(i)
Given that when 18 is added to the number, the digits are interchanged
∴(10x+y)+18=(10y+x)
⇒9x−9y+18=0
⇒9(x−y+2)=0
⇒x−y+2=0
⇒
y
35
−y+2=0{from (i)}
⇒35−y
2
+2y=0
⇒y
2
−2y−35=0
⇒(y+5)(y−7)=0
∵y
=−5
∴y=7
Substituting the value of y in (i), we get
x=
7
35
=5
Hence the two digit no. is 57.
-
Author:
earllyons
-
Rate an answer:
8
The Nos are 25 and 21
Explanation is in the pic
-
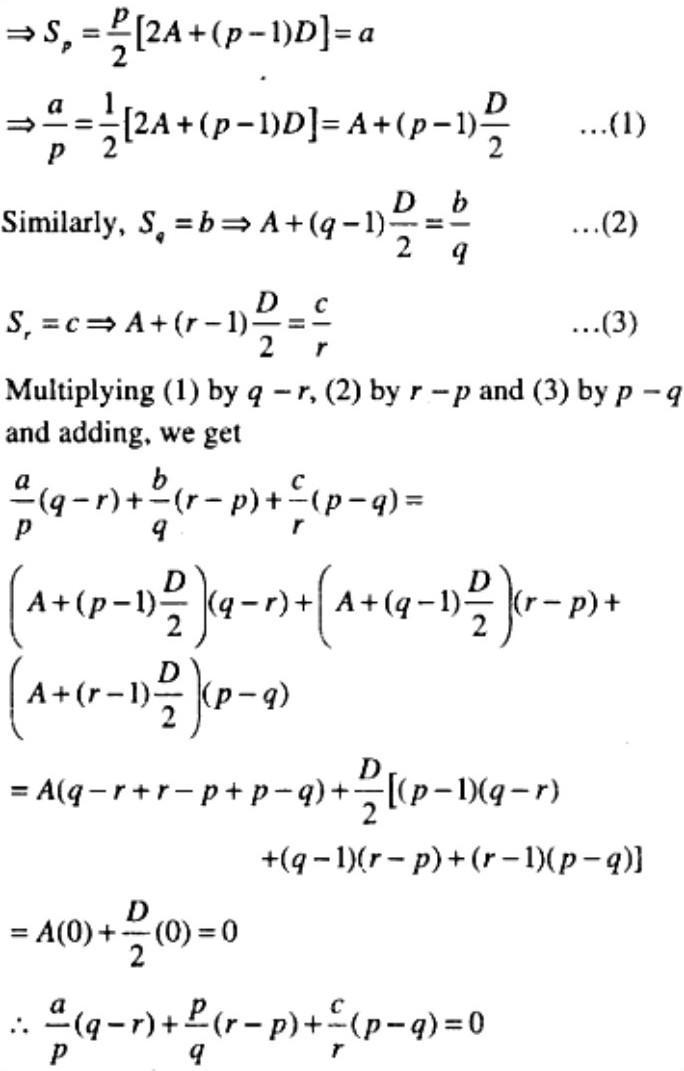
Author:
dobie
-
Rate an answer:
10
If you know the answer add it here!
Other related questions that may help you
-
Author:
davidr0wd
-
Author:
blimpiedptd
-
Rate an answer:
14
-
Author:
maddendalton
-
Rate an answer:
0
