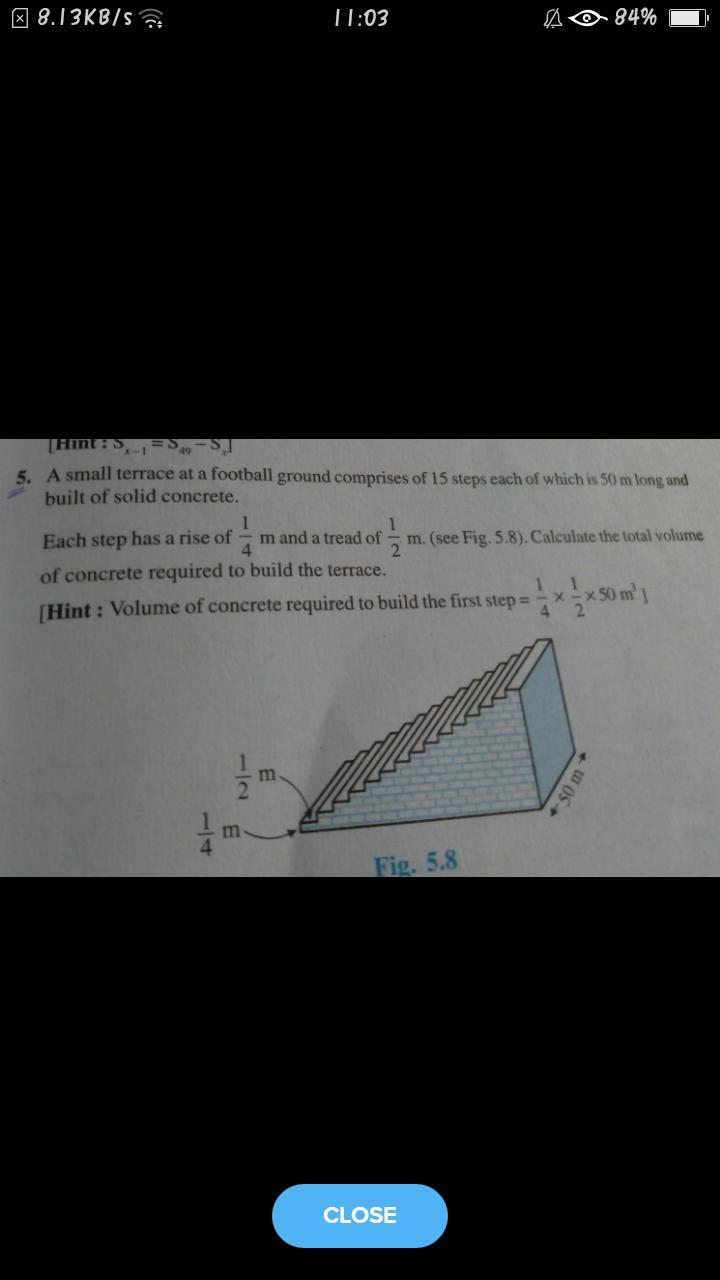
A small terrace at a football ground comprises of 15 steps each of which is 50m long and built of solid concrete. Each step has a rise of ¼ m and a tread of ½ m (See figure) calculate the total volume of concrete required to build the terrace.
-
Subject:
Math -
Author:
deannaatkinson -
Created:
1 year ago
Answers 2
Answer: 750m
Step-by-step explanation:
From the figure, it can be observed that the Height of 1st step is ¼ m
Height of the 2nd step is (¼ + ¼) m = ½ m
Height of 3rd step is (¼ + ¼ + ¼) m = 3/4 m
Therefore, the height of each step is increasing by ¼ m
Length 50m and width (tread) is the same for each step that is ½ m
Volume of step can be considered as Volume of Cuboid = length × breadth × height
Volume of concrete in 1st step = 50m × ½ m × ¼ m = 6.25 m³
Volume of concrete in 2nd step = 50m × ½ m × ½ m = 12.50 m3
Volume of concrete in 3rd step = 50m × ½ m × ¾ m = 18.75 m3
It can be observed that the volumes of concrete in these steps are in an A.P.: 6.25m³, 12.50m³, 18.75m³, ....
First term a = 6.25, Common difference d = 6.25
Let nth term of AP be the 15th value since number of steps = 15
Sum of n terms, Sₙ = n/2 [2a + (n - 1) d]
S₁₅ = 15 / 2 [2 × 6.25 + (15 - 1) × 6.25]
= 15 / 2 [12.50 + 14 × 6.25]
= 15 / 2 [12.50 + 87.50]
= 15/2 × 100
= 750 m³
Therefore, the volume of concrete required to build the terrace is 750 m³.
-
Author:
olivialowery
-
Rate an answer:
7
[tex]\large\underline{\sf{Solution-}}[/tex]
Given that,
A small terrace at a football ground comprises of 15 steps each of which is 50 m long and built of solid concrete. Each step has a rise of ¼ m and a tread of ½ m.
It means,
Length of each step, l = 50 m
Breadth of each step, b = \dfrac{1}{2} m
Let assume that h represents the height of first step and h = \dfrac{1}{4} m
Now,
[tex] \red{\sf \:Volume\:of\:concrete\:used\:in\:first\:step, \: V_1 = lbh \: {m}^{3} }\\ \\ [/tex]
[tex] \red{\sf \:Volume\:of\:concrete\:used\:in\: {2}^{nd} \:step, \: V_2 = lb(2h) = 2lbh \: {m}^{3} }\\ \\ [/tex]
[tex] \red{\sf \:Volume\:of\:concrete\:used\:in\: {3}^{rd} \:step, \: V_3 = lb(3h) = 3lbh \: {m}^{3} }\\ \\ [/tex]
.
.
.
.
.
[tex] \red{\sf \:Volume\:of\:concrete\:used\:in\: {15}^{th} \:step, \: V_{15} = lb(15h) = 15lbh \: {m}^{3} }\\ \\ [/tex]
So, Total volume of concrete required to built the terrace is
[tex] \sf \: = \: V_1 + V_2 + V_3 + \cdots \cdots \: + \: V_{15} \\ \\ [/tex]
[tex] \sf \: = \: lbh + 2lbh + 3lbh + \cdots \cdots \: + \: 15lbh \\ \\ [/tex]
[tex] \sf \: = \: lbh \: (1 + 2 + 3 + \cdots \cdots \: + \: 15) \\ \\ [/tex]
We know,
[tex]\boxed{ \bf{ \:1 + 2 + 3 + \cdots \cdots + n = \frac{n(n + 1)}{2} \: }} \\ \\ [/tex]
So, using this result and also on substituting the values of l, b and h, we get
[tex] \sf \: = \: 50 \times \dfrac{1}{2} \times \dfrac{1}{4} \times \bigg(\dfrac{15(15 + 1)}{2} \bigg) \\ \\ [/tex]
[tex] \sf \: = \: 25 \times \dfrac{1}{4} \times \bigg(\dfrac{15 \times 16}{2} \bigg) \\ \\ [/tex]
[tex] \sf \: = \: 25 \times 15 \times 2 \\ \\ [/tex]
[tex] \sf \: = \: 750 \: {cm}^{3} \\ \\ [/tex]
[tex]\bf\implies \:Volume\:of\:concrete\: = 750 \: {m}^{3} \\ \\ [/tex]
[tex]\rule{190pt}{2pt} \\ [/tex]
[tex] { \red{ \mathfrak{Additional\:Information}}}[/tex]
↝ nᵗʰ term of an arithmetic sequence is,
[tex]\begin{gathered}\red\bigstar\:\:{\underline{\orange{\boxed{\bf{\green{a_n\:=\:a\:+\:(n\:-\:1)\:d}}}}}} \\ \end{gathered}[/tex]
Wʜᴇʀᴇ,
aₙ is the nᵗʰ term.
a is the first term of the sequence.
n is the no. of terms.
d is the common difference.
[tex] \\[/tex]
↝ Sum of n terms of an arithmetic sequence is,
[tex]\begin{gathered}\red\bigstar\:\:{\underline{\orange{\boxed{\bf{\green{S_n\:=\dfrac{n}{2} \bigg(2 \:a\:+\:(n\:-\:1)\:d \bigg)}}}}}} \\ \end{gathered}[/tex]
Wʜᴇʀᴇ,
Sₙ is the sum of n terms of AP.
a is the first term of the sequence.
n is the no. of terms.
d is the common difference.
-
Author:
ortegaic1k
-
Rate an answer:
13
