Saurology is the study of ___________. (A) Mosquitoes (B) Snake (C) Lizards (D) Cockroach​

Answer

AnswerMy Diary

AnswerMy DiaryThe only friend I have ever had that has never fought, never demanded, never interrupted and always listened patiently without advising-my diary.

AnswerMy DiaryThe only friend I have ever had that has never fought, never demanded, never interrupted and always listened patiently without advising-my diary.We became friends when I was in the seventh grade but then, I was just using her to add to my sense of style. By the time I was in grade nine, we were inseparable. I could not do without her. A stage had come when she was the only one who had a right over my secrets. I betrayed my family secrets to her occasionally, as well- “Mom has been partial to my brother again just because he is back from me

Answer:

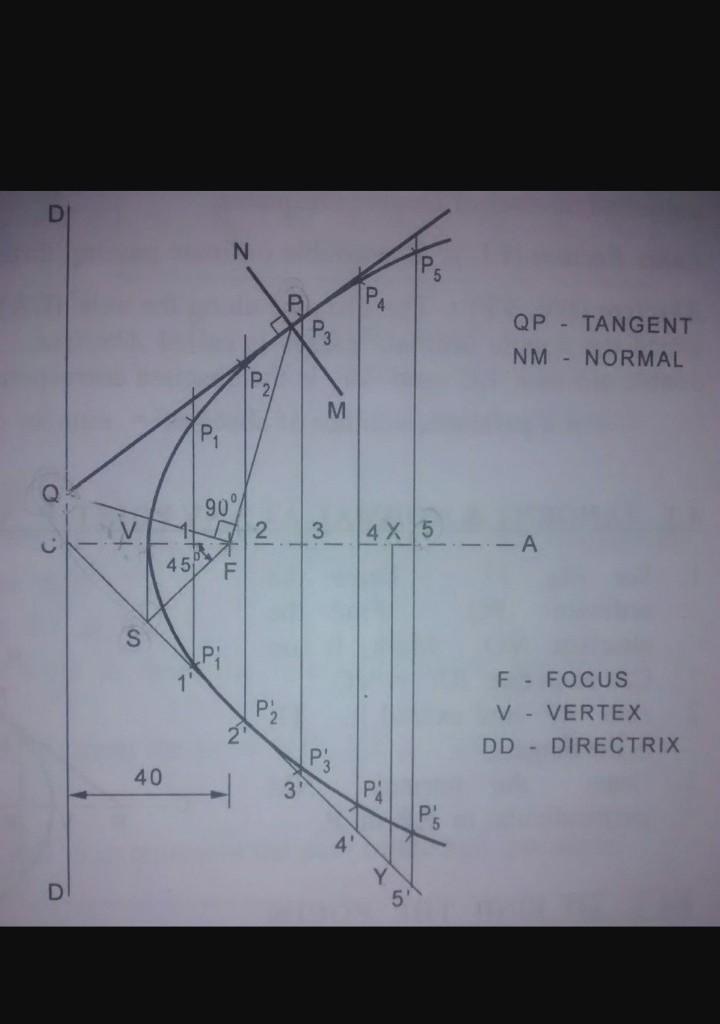
. In figure 1, point A is constructed as the focus and line BD as the directrix.

Figure 1

2. Next, point C is randomly constructed on segment BD. Through C, a line perpendicular to segment BD is constructed. Point C must be constructed randomly to insure that the perpendicular line can move along segment BD.

Figure 2

3. Now, segment AC is constructed with its perpendicular bisector line FE.

Figure 3

To understand this step, it might be helpful to keep in mind the definition of the parabola and to think about its vertex. Its vertex will be located at point E halfway between line BD and point A when the perpendicular is in line with point A. (See figure 4.)

Figure 4

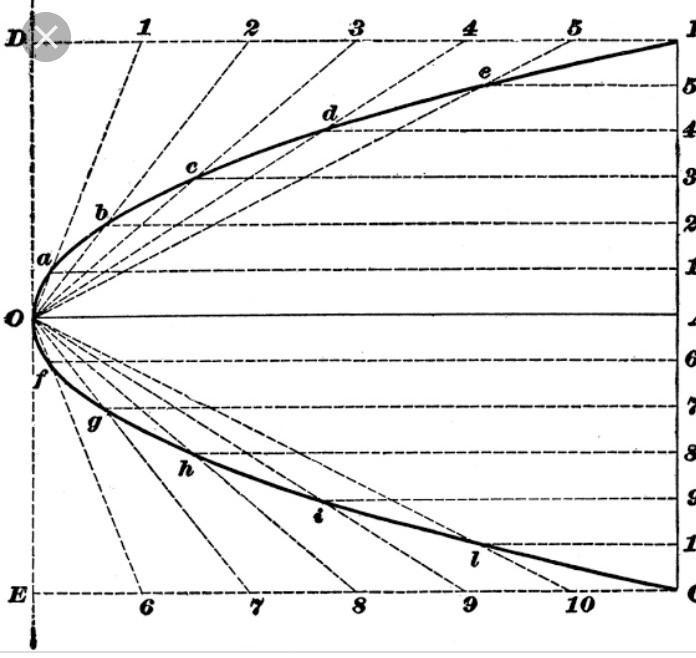
4. Next, select point F, choose “trace” from the Display Menu and then drag point C back and forth along segment BD to draw the parabola as shown in figure 5. (The more you “trace” the parabola the darker it becomes.)

Figure 5

To download a GSP animation of figure 5, go here and select “Animate Point.”

5. Geometer’s Sketchpad allows for two alternate constructions of parabolas as well.

a. The parabola in figure 6 has been created by tracing the perpendicular line FE as point C is dragged along segment BD.

Figure 6

b. The parabolas in figures 7 and 8 have been constructed using the locus command in the Construct Menu. (Figure 7 uses points C and F and figure 8 uses point C and line FE.) Note that this command yields the same results found above.

Figure 7

Figure 8

Connections to the other Conic Sections

6. What if the directrix was a circle instead of a line?

a. Consider figure 9 when point A is outside circle O and the line perpendicular to segment AB has been selected to trace. When point B is moved around the circle, a hyperbola is created.

Figure 9

b. Now consider figure 10 when point A is inside the circle. When point B is moved around the circle, an ellipse is created.

Figure 10

follow this attachment

Answer:

1. व्यवहार की वह रीति जिससे अपना कल्याण हो और समाज को भो कोई बाधा न पहुँचे ।
2. क्षमताशाली । 2. काम करने के योग्य । कार्य में समर्थ ।
3. अमूल्य संस्कृत [विशेषण] 1. जिसका मूल्य न हो ; अनमोल 2. बहुमूल्य ; मूल्यवान ; बेशकीमती 3. श्रेष्ठ ; उत्तम।

Explanation:

Answer:

व्यवहार की वह रीति जिससे अपना कल्याण हो और समाज को भो कोई बाधा न पहुँचे ।

क्षमताशाली । 2. काम करने के योग्य । कार्य में समर्थ ।

अमूल्य संस्कृत [विशेषण] 1. जिसका मूल्य न हो ; अनमोल 2. बहुमूल्य ; मूल्यवान ; बेशकीमती 3. श्रेष्ठ ; उत्तम।

Answer:

ಇಂದಿನ ದಿನದಲ್ಲಿ ಮೊಬೈಲ್ ಫೋನ್‌ಗಳು ಜೀವನದ ಬಹುಮುಖ್ಯ ಭಾಗವಾಗಿ ಮಾರ್ಪಟ್ಟಿದೆ. ಬರಿಯ ಕರೆ ಮಾತ್ರವಲ್ಲದೆ ಸಂದೇಶ ರವಾನೆ, ಗೇಮ್ಸ್ ಆಡಲು, ಅಪ್ಲಿಕೇಶನ್‌ಗಳನ್ನು ಬಳಸಿಕೊಳ್ಳಲು, ಇಮೇಲ್, ಚಾಟಿಂಗ್, ಹೀಗೆ ನಿಮ್ಮ ಅಂಗೈಯಗಲದ ಮಾಣಿಕ್ಯ ಹತ್ತು ಹಲವು ಬಗೆಯಲ್ಲಿ ವರವಾಗಿ ಪರಿಣಮಿಸಿದೆ. ಓದಿರಿ: ಸ್ಮಾರ್ಟ್‌ಫೋನ್ ಅನ್ನು ವೇಗವಾಗಿ ಚಾರ್ಜ್ ಮಾಡುವ ಟಿಪ್ಸ್ ಇಲ್ಲಿದೆ ಅತಿ ಆದರೆ ಅಮೃತ ಕೂಡ ವಿಷ ಎಂಬ ಮಾತಿನಂತೆ ಬಹುಪಯೋಗಿ ಸ್ಮಾರ್ಟ್‌ಫೋನ್ ನಿಮಗೆ ಪ್ರಯೋಜನಕಾರಿ ಹೇಗೆಯೋ ಅಂತೆಯೇ ನಿಮ್ಮ ಜೀವನಕ್ಕೆ ಸಂಚಕಾರವನ್ನು ತರಬಲ್ಲವು. ಅದು ಹೇಗೆ ಎಂಬುದನ್ನು ಅರಿತುಕೊಳ್ಳಲು ಈ ಲೇಖನ ನಿಮಗೆ ಸಹಕಾರಿಯಾಗಿದೆ. ನಿಮ್ಮ ಡಿವೈಸ್ ಅನ್ನು ನೀವು

ಬಹುವಾಗಿ ಬಳಸುತ್ತಿದ್ದಲ್ಲಿ ಈ ಲೇಖನ ನಿಮಗೆ ಇದರ

ಅಪಾಯಗಳನ್ನು ತಿಳಿಸಿಕೊಡಲಿದೆ.