Find the remainder when a polynomial p(x)=r³-6r² + 2x-4 is divided by (3x-1).
Answers 2
Step-by-step explanation:
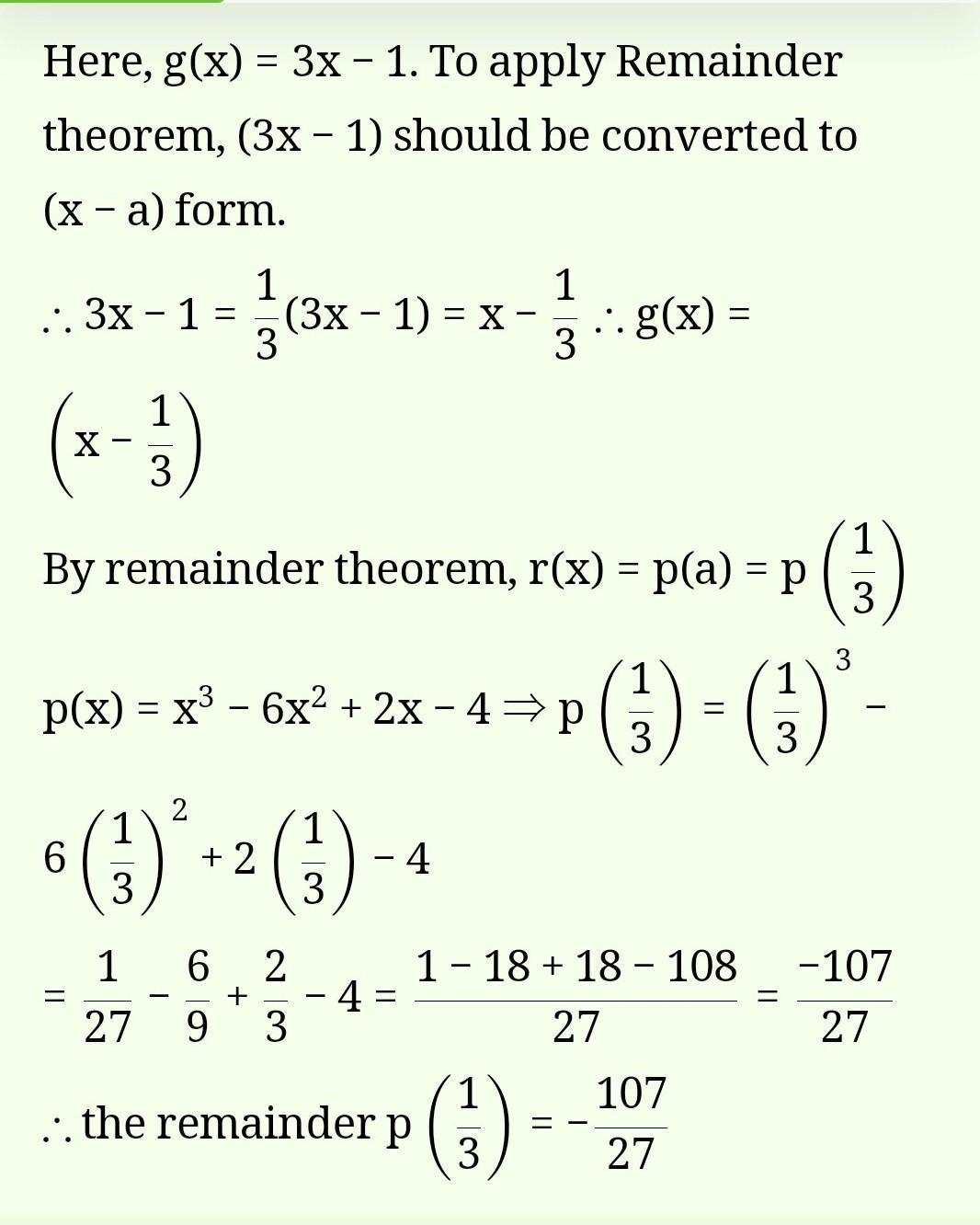
Here, g(x)=3x−1. To apply Remainder theorem, (3x−1) should be converted to (x−a) form.
∴3x−1=
3
1
(3x−1)=x−
3
1
∴g(x)=(x−
3
1
)
By remainder theorem, r(x)=p(a)=p(
3
1
)
p(x)=x
3
−6x
2
+2x−4⇒p(
3
1
)=(
3
1
)
3
−6(
3
1
)
2
+2(
3
1
)−4
=
27
1
−
9
6
+
3
2
−4=
27
1−18+18−108
=
27
−107
∴ the remainder p(
3
1
)=−
27
107
-
Author:
wizygbw
-
Rate an answer:
5
here, g(x)=3x-1. To apply remainder theorem, (3x-1) should be converted to (x-a) form
3x-1= 1 by 3 (3x-1) = x- 1 by 3
g(x) =(x-1 by 3)
by remainder theorem,
r(x) = p(a) =p(1 by 3)
p(x)=x3 - 6x2 + 2x - 4 =p(1 by 3)
= (1 by 3)3 - 6(1 by 3)2 + 2(1 by 3 ) - 4
= 1 by 27 - 6 by 9 + 2 by 3 - 4
= 1-18+18-108 by 27
= -107 by 27
Therefore, the remainder is -107 by 27
-
Author:
yoselinmnnp
-
Rate an answer:
9
