Answer fast pls pls dont spam

-
Subject:
Math -
Author:
kaseyhurley -
Created:
1 year ago
Answers 2
Step-by-step explanation:
sorry ,I don't know 2 answer ..
please mark me as brainlist.

-
Author:
lovey23
-
Rate an answer:
6
Answer:
see i am not knowing how to do question 20 so skipping it i will do rest of the two,
hope these two which i am answering will help you
and i am sorry for not solving all three
Step-by-step explanation:
i know i have done many cutting so for that i am leaving the solution here also
Q19.let the angles be x
1x+2x+3x=180 (by angle sum property)
6x=180
x=180/6
x=30
1x= 30
2x=60
3x=90
Q20.[tex]rationalising \: \frac{ \sqrt{5} }{ \sqrt{6 } + 2} [/tex]
[tex] \frac{ \sqrt{5} }{ \sqrt{6} + 2} \times \frac{ \sqrt{6} - 2}{ \sqrt{6} - 2} [/tex]
[tex] \frac{ \sqrt{30} - 2 \sqrt{5} }{ { \sqrt{6} }^{2} - {2}^{2} } [/tex]
[tex] \frac{ \sqrt{30 } - 2 \sqrt{5} }{6 - 4} = > \frac{ \sqrt{30} - 2 \sqrt{5} }{2} [/tex]
[tex]rationalising \: \frac{ \sqrt{5} }{ \sqrt{6} - 2} [/tex]
[tex] \frac{ \sqrt{5} }{ \sqrt{6} - 2} \times \frac{ \sqrt{6} - 2}{ \sqrt{6} - 2} = > \frac{ \sqrt{30} + 2 \sqrt{5} }{ { \sqrt{6} }^{2} - {2}^{2} } [/tex]
[tex] \frac{ \sqrt{30} + 2 \sqrt{5} }{6 - 4} = > \frac{ \sqrt{30} + 2 \sqrt{5} }{2} [/tex]
[tex]now \: simplifying[/tex]
[tex] \frac{ \sqrt{30} - 2 \sqrt{5} }{2} - \frac{ \sqrt{30} + 2 \sqrt{5} }{2} \\ = > \frac{ \sqrt{30} - 2 \sqrt{5} - \sqrt{30} + 2 \sqrt{5} }{2} [/tex]
[tex] \frac{1}{2} answer[/tex]
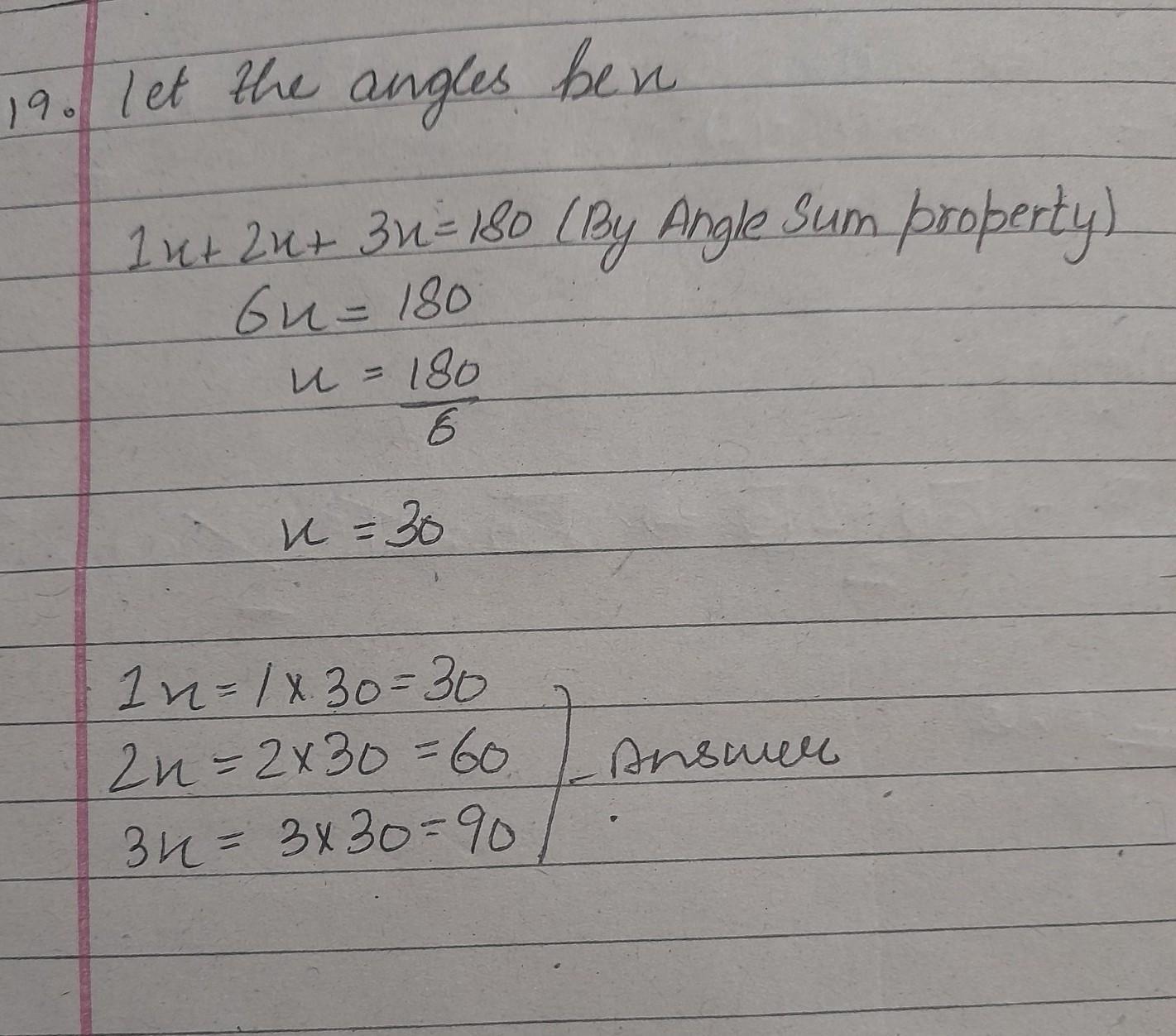

-
Author:
willoqsv
-
Rate an answer:
14
