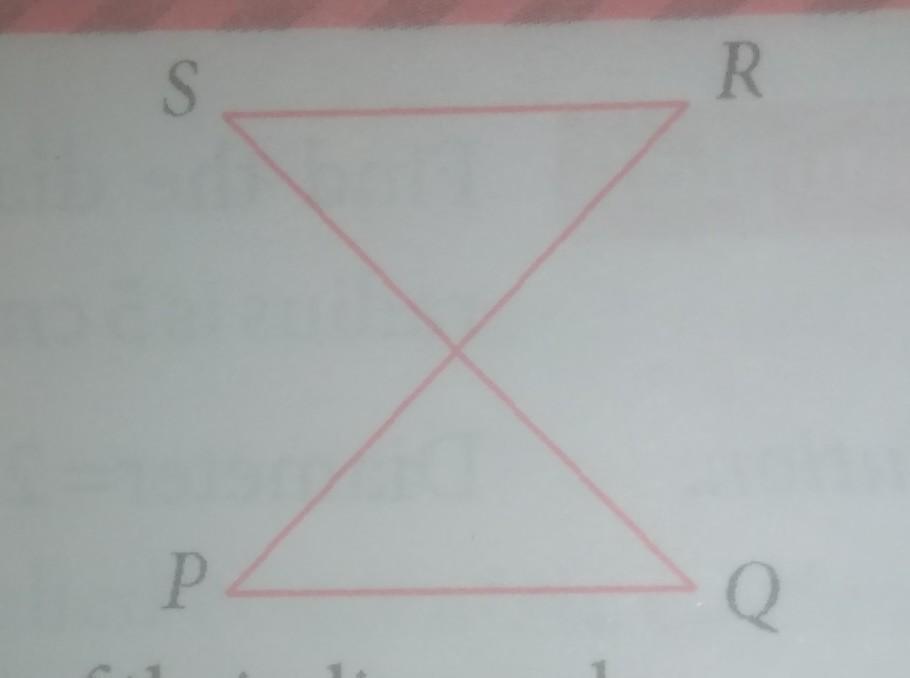
Is the adjoining figure a quadrilateral ? give reasons supporting your answer
Answers 2
Answer:
No, the figure is not quadrilateral because they are not joined as you can see in this pic.
-
Author:
josefamccoy
-
Rate an answer:
9
Answer:
No, this is not quadrilaterals
Step-by-step explanation:
hope it help you
-
Author:
leiawhitney
-
Rate an answer:
13
If you know the answer add it here!
Choose a language and a region
How much to ban the user?
1 hour
1 day
100 years