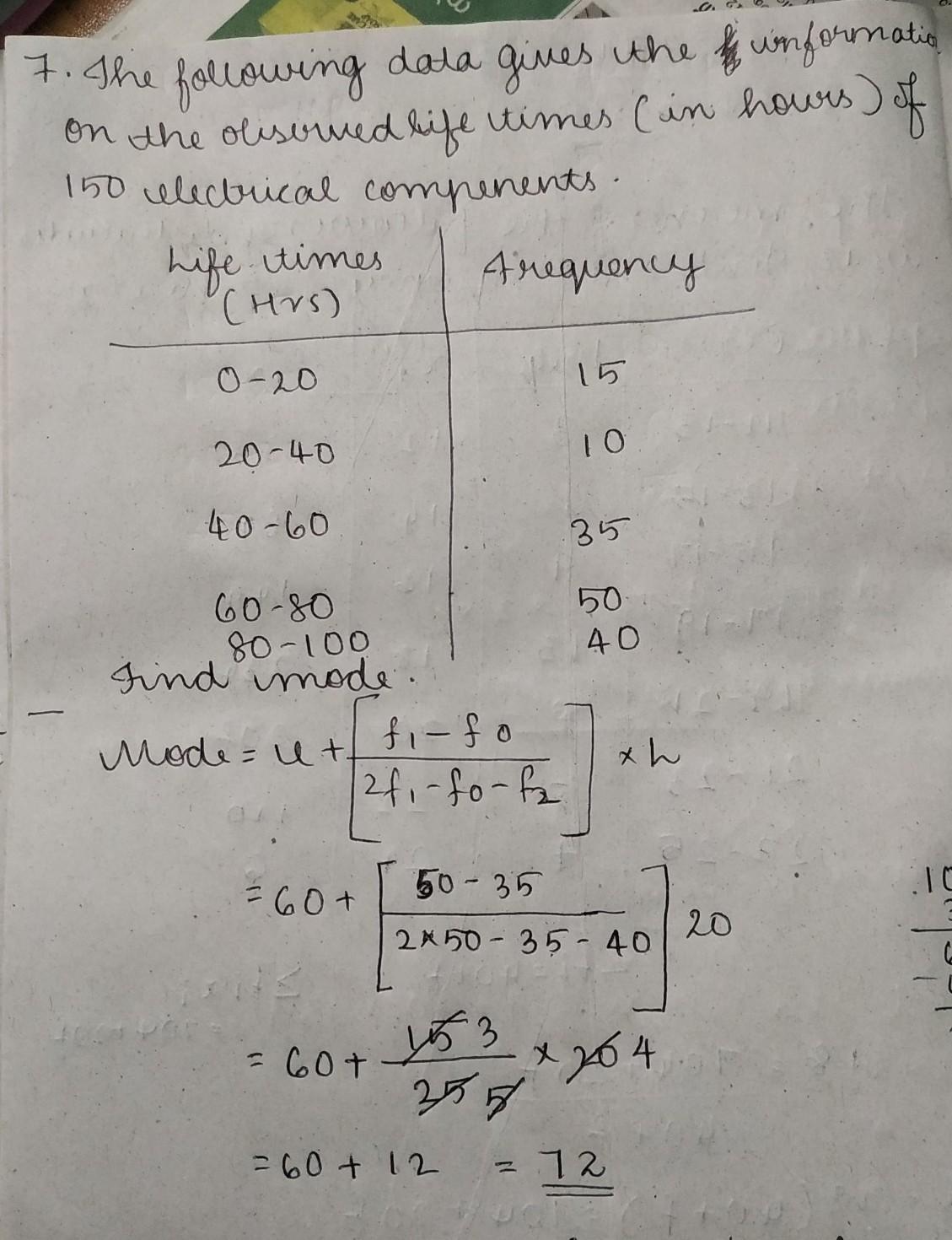
3. The following data gives the information on the observed life times in hours) of 150 components electrical components Life Time (in hours) 0-20 20 - 40 40 - 60 60 - 80 80 - 100 Frequency 15 10 35 50 40 Find the mode of distribution.

Answers 2

Answer:

Mode= 72

Hope this helps!

Answer:

mode of the given grouped data = 72

Step-by-step explanation:

class frequency

0-20 15

20-40 10

40-60 35

60-80 50

80-100 40

maximum frequency is 50 and the corresponding class is 60-80

so modal class is 60-80

for a grouped data, mode = [tex]l+\frac{(f1-fo)h}{2f1-fo-f2}[/tex]

where:

l is the lower limit of the modal class = 60

fo is the frequency anteceding the modal class = 35

f1 is the frequency of the modal class = 50

f2 is the frequency following the modal class = 40

and, h is the size of class interval = 20

then: mode = 60 + [tex]\frac{(50-35)20}{2(50) - 35-40}[/tex]

⇒ mode = 60 + [tex]\frac{(15)(20)}{25}[/tex]

= 60 + 12

= 72

hence, mode of the given grouped data = 72