1. A farmer connects a pipe of internal diameter 25 cm from a canal into a cylindrical tank in his field, which is 12 m in diameter and 2.5 m deep. If water flows through the pipe at the rate of 3.6 km/hr, in how much time will the tank be filled? Also, find the cost of water if the canal department charges at the rate of 0.07 per mº. [CBSE 2009C] 17
Answers 2
Answer:
Radius (r
1
) of circular end of pipe =
200
20
=0.1 m
⇒Area of cross-section =π×r
1
2
=π×(0.1)
2
=0.01π sq. m
⇒Speed of water =3 kilometer per hour =
60
3000
=50 meter per minute.
⇒Volume of water that flows in 1 minute from pipe = 50×0.01π=0.5π cu. m
⇒From figure 2, Volume of water that flows in t minutes from pipe = t×0.5π cu. m
⇒Radius (r
2
) of circular end of cylindrical tank =
2
10
=5 m
⇒Depth (h
2
) of cylindrical tank =2 m
⇒Let the tank be filled completely in t minutes.
⇒The volume of water filled in tank in t minutes is equal to the volume of water flowed in t minutes from the pipe.
⇒Volume of water that flows in t minutes from pipe = Volume of water in tank
Therefore, t×0.5π=πr
2
2
×h
2
⇒t×0.5=5
2
×2
⇒t=
0.5
25×2
⇒t=100
Therefore, the cylindrical tank will be filled in 100 minute.
-
Author:
kittyhood
-
Rate an answer:
11
Answer:
That is your answer in attachment.
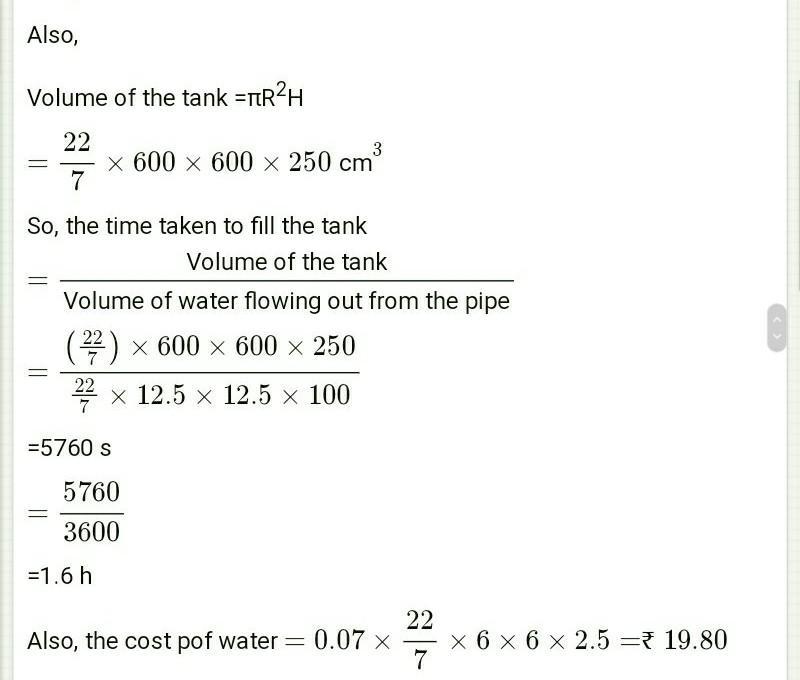
-
Author:
daniellamk03
-
Rate an answer:
8
