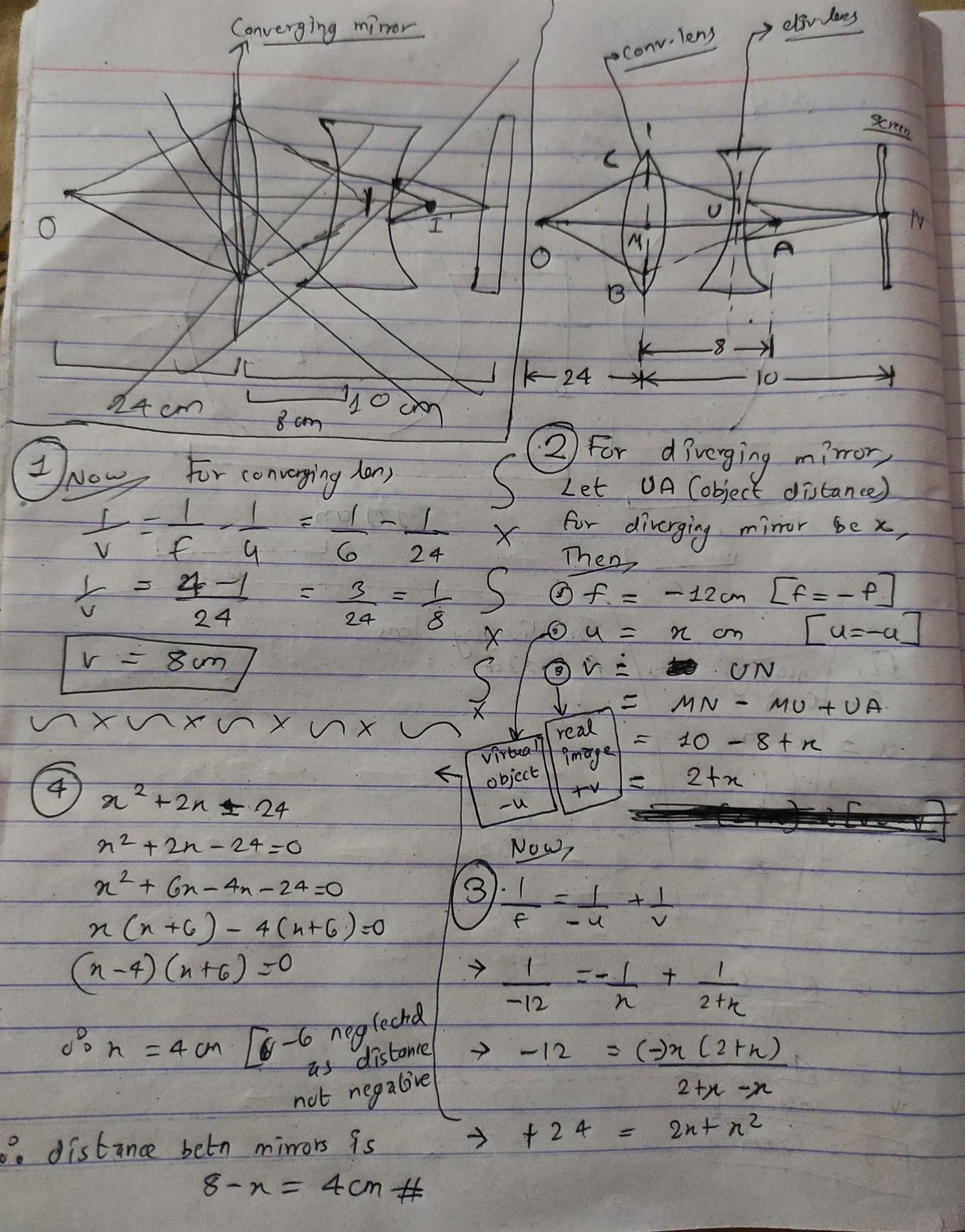
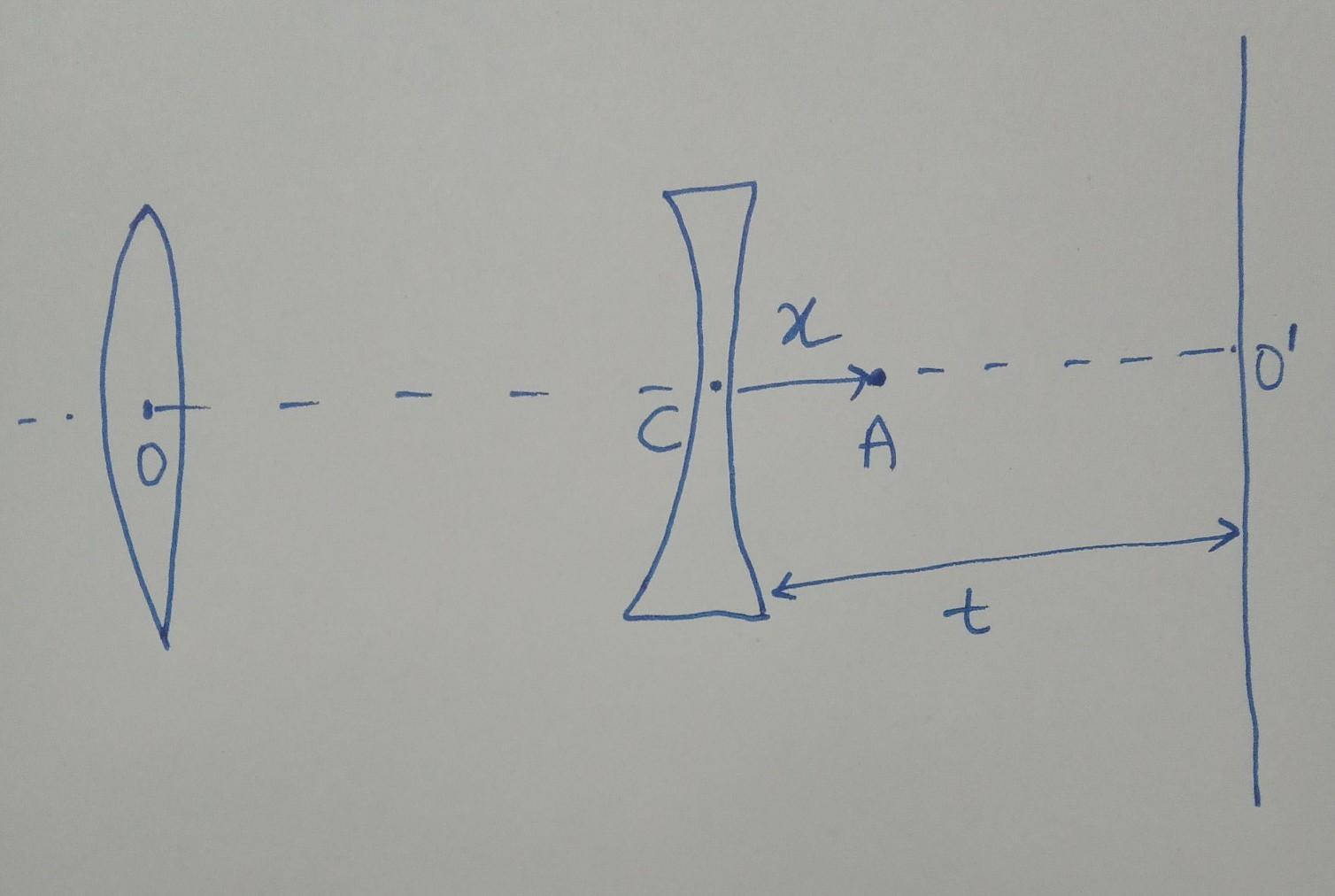
Adhikansh paudhon Mein Anda Se viksit Hota Hai
-
Subject:
Biology -
Author:
isailozano -
Created:
1 year ago
Answers 1
Answer:
अधिकांश पौधों में, जब तक अंडाशय से फल विकसित होता है, तब तक अन्य पुष्प भाग पतित हो जाते हैं और गिर जाते हैं। हालाँकि, कुछ प्रजातियों जैसे सेब, स्ट्रॉबेरी, काजू, आदि में, थैलेमस भी फलों के निर्माण में योगदान देता है। ऐसे फल कूट फल कहलाते हैं।
-
Author:
grant298
-
Rate an answer:
6
If you know the answer add it here!
Choose a language and a region
How much to ban the user?
1 hour
1 day
100 years